Quadratics and Polynomials Word Problems Review Question 6 Answers
14.vi: Polynomial Equations
- Page ID
- 46379
Past the stop of this department, yous will be able to:
- Use the Zero Product Property
- Solve quadratic equations by factoring
- Solve equations with polynomial functions
- Solve applications modeled by polynomial equations
Before you get started, take this readiness quiz.
- Solve: \(5y−3=0\).
If you missed this trouble, review [link]. - Factor completely: \(n^3−9n^ii−22n\).
If you lot missed this problem, review [link]. - If \(f(x)=8x−xvi\), find \(f(3)\) and solve \(f(x)=0\).
If you lot missed this problem, review [link].
We have spent considerable time learning how to factor polynomials. We will now look at polynomial equations and solve them using factoring, if possible.
A polynomial equation is an equation that contains a polynomial expression. The degree of the polynomial equation is the degree of the polynomial.
A polynomial equation is an equation that contains a polynomial expression.
The caste of the polynomial equation is the degree of the polynomial.
We have already solved polynomial equations of degree ane. Polynomial equations of caste ane are linear equations are of the form \(ax+b=c\).
We are now going to solve polynomial equations of degree two. A polynomial equation of degree two is chosen a quadratic equation. Listed below are some examples of quadratic equations:
\[x^2+5x+6=0 \qquad 3y^2+4y=x \qquad 64u^2−81=0 \qquad n(due north+1)=42 \nonumber\]
The last equation doesn't appear to have the variable squared, but when we simplify the expression on the left we volition become \(northward^2+n\).
The general course of a quadratic equation is \(ax^two+bx+c=0\), with \(a\neq 0\). (If \(a=0\), then \(0·x^2=0\) and we are left with no quadratic term.)
An equation of the course \(ax^2+bx+c=0\) is called a quadratic equation.
\[a,b,\text{ and }c\text{ are real numbers and }a\neq 0\nonumber\]
To solve quadratic equations we demand methods dissimilar from the ones we used in solving linear equations. We will expect at 1 method here and then several others in a later chapter.
Employ the Nothing Product Property
Nosotros will first solve some quadratic equations by using the Zero Production Property. The Cypher Product Property says that if the product of two quantities is zero, and so at least one of the quantities is zero. The merely mode to become a product equal to zero is to multiply by zip itself.
If \(a·b=0\), then either \(a=0\) or \(b=0\) or both.
We will now utilise the Zero Product Property, to solve a quadratic equation.
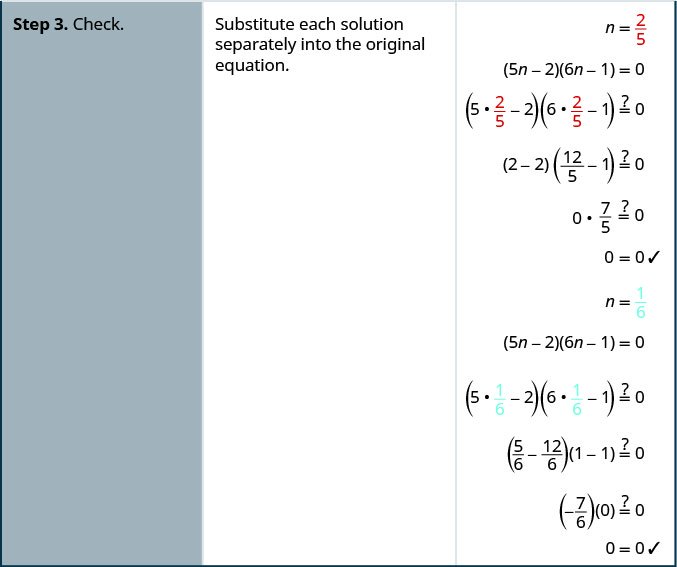
Solve: \((5n−2)(6n−one)=0\).
- Answer
-


Solve: \((3m−2)(2m+one)=0\).
- Answer
-
\(m=\frac{2}{3},\space m=−\frac{1}{2}\)
Solve: \((4p+iii)(4p−iii)=0\).
- Answer
-
\(p=−\frac{three}{4},\space p=\frac{3}{4}\)
- Set up each factor equal to aught.
- Solve the linear equations.
- Cheque.
Solve Quadratic Equations by Factoring
The Zero Product Belongings works very nicely to solve quadratic equations. The quadratic equation must be factored, with cypher isolated on 1 side. So we be sure to showtime with the quadratic equation in standard course, \(ax^2+bx+c=0\). And then we factor the expression on the left.
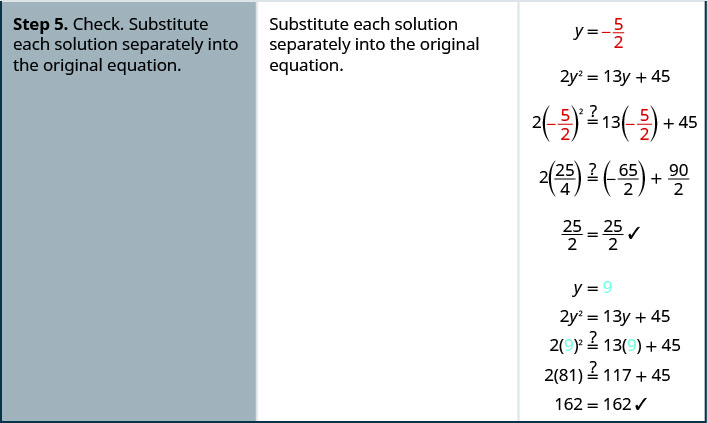
Solve: \(2y^2=13y+45\).
- Answer
-




Solve: \(3c^2=10c−viii\).
- Answer
-
\(c=2,\infinite c=\frac{4}{3}\)
Solve: \(2d^2−5d=3\).
- Reply
-
\(d=3,\space d=−12\)
- Write the quadratic equation in standard grade, \(ax^ii+bx+c=0\).
- Factor the quadratic expression.
- Use the Zero Product Property.
- Solve the linear equations.
- Check. Substitute each solution separately into the original equation.
Before nosotros factor, we must brand sure the quadratic equation is in standard class.
Solving quadratic equations by factoring volition brand use of all the factoring techniques you lot have learned in this affiliate! Do you recognize the special product pattern in the next example?
Solve: \(169q^ii=49\).
- Respond
-
\(\begin{assortment} {ll} &169x^two=49 \\ \text{Write the quadratic equation in standard class.} &169x^2−49=0 \\ \text{Cistron. It is a difference of squares.} &(13x−7)(13x+7)=0 \\ \text{Utilize the Goose egg Product Holding to set each factor to }0. & \\ \text{Solve each equation.} &\brainstorm{array} {ll} 13x−vii=0 &13x+7=0 \\ 13x=seven &13x=−seven \\ 10=\frac{seven}{13} &10=−\frac{7}{thirteen} \cease{array} \end{array}\)
Check:
Nosotros get out the check up to you.
Solve: \(25p^2=49\).
- Answer
-
\(p=\frac{7}{5},p=−\frac{7}{v}\)
Solve: \(36x^2=121\).
- Answer
-
\(ten=\frac{eleven}{vi},10=−\frac{11}{six}\)
In the next example, the left side of the equation is factored, but the correct side is not nix. In order to use the Zero Production Belongings, ane side of the equation must be nothing. Nosotros'll multiply the factors and and so write the equation in standard form.
Solve: \((3x−8)(x−ane)=3x\).
- Answer
-
\(\brainstorm{assortment} {ll} &(3x−8)(x−one)=3x \\ \text{Multiply the binomials.} &3x^2−11x+viii=3x \\ \text{Write the quadratic equation in standard form.} &3x^2−14x+8=0 \\ \text{Factor the trinomial.} &(3x−2)(x−4)=0 \\ \brainstorm{assortment} {l} \text{Use the Zero Product Holding to set each cistron to 0.} \\ \text{Solve each equation.} \end{array} &\begin{array} {ll} 3x−2=0 &x−four=0 \\ 3x=two &ten=4 \\ ten=\frac{two}{3} & \terminate{assortment} \\ \text{Cheque your answers.} &\text{The check is left to you.} \cease{assortment}\)
Solve: \((2m+1)(m+3)=12m\).
- Answer
-
\(one thousand=1,\space m=\frac{three}{two}\)
Solve: \((thousand+1)(k−1)=eight\).
- Respond
-
\(k=iii,\space k=−3\)
In the side by side example, when nosotros factor the quadratic equation we will get three factors. However the starting time factor is a abiding. We know that factor cannot equal 0.
Solve: \(3x^ii=12x+63\).
- Answer
-
\(\begin{array} {ll} &3x^ii=12x+63 \\ \text{Write the quadratic equation in standard form.} &3x^2−12x−63=0 \\ \text{Factor the greatest common factor first.} &iii(x^2−4x−21)=0 \\ \text{Gene the trinomial.} &three(10−7)(x+3)=0 \\ \begin{array} {l} \text{Use the Zero Production Property to set each factor to 0.} \\ \text{Solve each equation.} \end{array} &\begin{array} {lll} 3\neq 0 &10−7=0 &x+iii=0 \\ three\neq 0 &ten=seven &x=−iii \finish{assortment} \\ \text{Check your answers.} &\text{The check is left to you.} \terminate{assortment}\)
Solve: \(18a^2−30=−33a\).
- Answer
-
\(a=−\frac{v}{2},a=\frac{ii}{iii}\)
Solve: \(123b=−6−60b^2\)
- Answer
-
\(b=−2,\space b=−\frac{1}{20}\)
The Nix Product Property also applies to the product of iii or more factors. If the product is zero, at to the lowest degree one of the factors must be zero. We tin can solve some equations of caste greater than two past using the Nothing Product Property, just similar we solved quadratic equations.
Solve: \(9m^3+100m=60m^2\)
- Answer
-
\(\brainstorm{array} {ll} & 9m^3+100m=60m^two \\ \text{Bring all the terms to one side so that the other side is zero.} &9m^three−60m^2+100m=0 \\ \text{Factor the greatest common factor first.} &m(9m^2−60m+100)=0 \\ \text{Factor the trinomial.} &m(3m−10)^ii=0 \end{array}\\ \begin{assortment} {l} \text{Apply the Zero Product Holding to fix each factor to 0.} \\ \text{Solve each equation.} &\brainstorm{array} {lll} thousand=0 &3m−ten=0 &{}\\ m=0 &m=\frac{10}{iii} & {} \end{array}\\ \text{Check your answers.} &\text{The check is left to yous.} \stop{assortment}\)
Solve: \(8x^3=24x^2−18x\).
- Reply
-
\(10=0,\space x=\frac{iii}{2}\)
Solve: \(16y^two=32y^3+2y\).
- Answer
-
\(y=0,\space y=14\)
Solve Equations with Polynomial Functions
As our study of polynomial functions continues, it will often be important to know when the part will have a certain value or what points lie on the graph of the part. Our work with the Zero Production Property will be assist united states of america find these answers.
For the part \(f(x)=x^2+2x−two\),
ⓐ find \(x\) when \(f(x)=six\)
ⓑ find two points that lie on the graph of the function.
- Answer
-
ⓐ
\(\brainstorm{array} {ll} &f(x)=x^2+2x−2 \\ \text{Substitute }half-dozen\text{ for }f(x). &6=x^2+2x−2 \\ \text{Put the quadratic in standard grade.} &x^ii+2x−8=0 \\ \text{Factor the trinomial.} &(x+4)(10−2)=0 \\ \brainstorm{assortment} {l} \text{Use the zero product property.} \\ \text{Solve.} \stop{assortment} &\begin{assortment} {lll} x+iv=0 &\text{or} &x−two=0 \\ x=−4 &\text{or} &10=two \end{array} \\ \text{Cheque:} & \\ & \\ & \\ & \\ & \\ & \\ & \\ & \\ & \\ \begin{array} {lll} \quad &\hspace{3mm} f(x)=x^2+2x−two &f(x)=10^2+2x−2 \\ \quad &f(−four)=(−iv)^2+2(−four)−2 &f(2)=2^2+2·2−2 \\ \quad &f(−4)=16−viii−ii &f(2)=4+4−2 \\ \quad &f(−four)=6\checkmark &f(2)=half-dozen\checkmark \end{array} & \end{assortment} \)ⓑ Since \(f(−4)=6\) and \(f(ii)=half dozen\), the points \((−four,6)\) and \((ii,6)\) prevarication on the graph of the function.
For the function \(f(x)=10^ii−2x−8\),
ⓐ find \(ten\) when \(f(x)=vii\)
ⓑ Find two points that prevarication on the graph of the function.
- Answer
-
ⓐ \(ten=−iii\) or \(10=5\)
ⓑ \((−iii,vii)\space (5,7)\)
For the part \(f(x)=ten^2−8x+3\),
ⓐ observe \(x\) when \(f(x)=−4\)
ⓑ Find two points that lie on the graph of the function.
- Reply
-
ⓐ \(x=1\) or \(x=vii\)
ⓑ \((1,−4)\space (vii,−four)\)
The Zero Product Property also helps us determine where the function is zero. A value of \(x\) where the function is \(0\), is chosen a zero of the function.
For whatever function \(f\), if \(f(10)=0\), and so \(x\) is a aught of the function.
When \(f(x)=0\), the indicate \((x,0)\) is a point on the graph. This signal is an \(x\)-intercept of the graph. It is ofttimes important to know where the graph of a function crosses the axes. We volition see some examples later.
For the function \(f(x)=3x^2+10x−8\), find
ⓐ the zeros of the function,
ⓑ whatsoever \(x\)-intercepts of the graph of the function
ⓒ any \(y\)-intercepts of the graph of the function
- Answer
-
ⓐ To detect the zeros of the function, we demand to find when the part value is 0.
\(\begin{assortment} {ll} &f(x)=3x^ii+10x−viii \\ \text{Substitute }0\text{ for}f(x). &0=3x^2+10x−eight \\ \text{Factor the trinomial.} &(x+four)(3x−2)=0 \\ \begin{array} {l} \text{Utilise the zero product property.} \\ \text{Solve.} \terminate{array} &\begin{array} {lll} ten+4=0 &\text{or} &3x−2=0 \\ x=−four &\text{or} &x=\frac{2}{3} \end{array} \end{array}\)ⓑ An \(x\)-intercept occurs when \(y=0\). Since \(f(−4)=0\) and \(f(\frac{2}{3})=0\), the points \((−4,0)\) and \((\frac{2}{3},0)\) prevarication on the graph. These points are \(ten\)-intercepts of the office.
ⓒ A \(y\)-intercept occurs when \(x=0\). To find the \(y\)-intercepts we demand to discover \(f(0)\).
\(\begin{array} {ll} &f(x)=3x^two+10x−eight \\ \text{Find }f(0)\text{ by substituting }0\text{ for }x. &f(0)=3·0^2+10·0−8 \\ \text{Simplify.} &f(0)=−viii \cease{array} \)
Since \(f(0)=−8\), the point \((0,−8)\) lies on the graph. This point is the \(y\)-intercept of the office.
For the part \(f(10)=2x^2−7x+5\), observe
ⓐ the zeros of the function
ⓑ whatever \(x\)-intercepts of the graph of the role
ⓒ any \(y\)-intercepts of the graph of the role.
- Respond
-
ⓐ \(x=one\) or \(x=\frac{v}{2}\)
ⓑ \((1,0),\space (\frac{5}{2},0)\) ⓒ \((0,5)\)
For the function \(f(x)=6x^2+13x−xv\), notice
ⓐ the zeros of the role
ⓑ whatever \(x\)-intercepts of the graph of the function
ⓒ whatever \(y\)-intercepts of the graph of the role.
- Answer
-
ⓐ \(x=−three\) or \(x=\frac{5}{6}\)
ⓑ \((−3,0),\infinite (\frac{5}{6},0)\) ⓒ \((0,−15)\)
Solve Applications Modeled by Polynomial Equations
The problem-solving strategy we used earlier for applications that translate to linear equations volition work just as well for applications that translate to polynomial equations. We volition copy the trouble-solving strategy hither so we can use it for reference.
- Read the problem. Brand certain all the words and ideas are understood.
- Identify what we are looking for.
- Proper name what we are looking for. Choose a variable to represent that quantity.
- Translate into an equation. It may exist helpful to recapitulate the trouble in one judgement with all the important data. So, translate the English judgement into an algebraic equation.
- Solve the equation using advisable algebra techniques.
- Check the answer in the problem and brand sure it makes sense.
- Respond the question with a complete sentence.
We volition start with a number trouble to go exercise translating words into a polynomial equation.
The production of ii sequent odd integers is 323. Find the integers.
- Respond
-
\(\begin{assortment} {ll} \textbf{Pace 1. Read }\text{the problem.} & \\ \textbf{Pace 2. Identify }\text{what nosotros are looking for.} &\text{We are looking for 2 consecutive integers.} \\ \textbf{Step 3. Name}\text{ what we are looking for.} &\text{Permit } n=\text{ the beginning integer.} \\ &n+two= \text{ side by side sequent odd integer} \\ \begin{assortment} {l} \textbf{Step iv. Interpret }\text{into an equation. Restate the}\hspace{20mm} \\ \text{problem in a sentence.} \stop{array} &\brainstorm{array} {l} \text{The production of the two consecutive odd} \\ \text{integers is }323. \stop{array} \\ &\quad n(n+2)=323 \\ \textbf{Step 5. Solve }\text{the equation.} north^2+2n=323 \\ \text{Bring all the terms to 1 side.} &n^2+2n−323=0 \\ \text{Factor the trinomial.} &(n−17)(n+xix)=0 \\ \begin{array} {l} \text{Employ the Nada Production Property.} \\ \text{Solve the equations.} \end{assortment} &\begin{array} {ll} north−17=0 \hspace{10mm}&n+nineteen=0 \\ n=17 &n=−nineteen \end{array} \stop{array} \)
At that place are 2 values for \(n\) that are solutions to this trouble. And then there are ii sets of consecutive odd integers that will work.\(\begin{assortment} {ll} \text{If the get-go integer is } northward=17 \hspace{60mm} &\text{If the first integer is } n=-xix \\ \text{then the next odd integer is} &\text{and so the next odd integer is} \\ \hspace{53mm} northward+ii &\hspace{53mm} due north+2 \\ \hspace{51mm} 17+2 &\hspace{51mm} -19+2 \\ \hspace{55mm} 19 &\hspace{55mm} -17 \\ \hspace{51mm} 17,19 &\hspace{51mm} -17,-19 \\ \textbf{Step half dozen. Check }\text{the answer.} & \\ \text{The results are consecutive odd integers} & \\ \begin{array} {ll} 17,\space nineteen\text{ and }−19,\space −17. & \\ 17·xix=323\checkmark &−xix(−17)=323\checkmark \end{array} & \\ \text{Both pairs of sequent integers are solutions.} & \\ \textbf{Step 7. Reply }\text{the question} &\text{The sequent integers are }17, xix\text{ and }−nineteen,−17. \terminate{assortment} \)
The production of ii consecutive odd integers is 255. Find the integers.
- Answer
-
\(−15,−17\) and \(15, 17\)
The production of 2 consecutive odd integers is 483 Find the integers.
- Answer
-
\(−23,−21\) and \(21, 23\)
Were y'all surprised by the pair of negative integers that is one of the solutions to the previous example? The product of the two positive integers and the production of the ii negative integers both requite positive results.
In some applications, negative solutions will event from the algebra, just will not be realistic for the situation.
A rectangular bedroom has an surface area 117 square feet. The length of the bedroom is four feet more than the width. Find the length and width of the bedroom.
- Answer
-
Footstep i. Read the problem. In problems involving
geometric figures, a sketch can help yous visualize
the state of affairs.
Step 2. Identify what you are looking for. We are looking for the length and width. Step 3. Name what y'all are looking for. Let \(w=\text{ the width of the bedchamber}\). The length is iv anxiety more than the width. \(w+4=\text{ the length of the garden}\) Step four. Translate into an equation. Restate the of import information in a sentence. The area of the bedroom is 117 square feet. Use the formula for the area of a rectangle. \(A=fifty·w\) Substitute in the variables. \(117=(west+four)w\) Stride 5. Solve the equation Distribute showtime. \(117=westward^2+4w\) Get zero on one side. \(117=westward^2+4w\) Factor the trinomial. \(0=w^2+4w−117\) Use the Zero Product Property. \(0=(w^two+thirteen)(westward−9)\) Solve each equation. \(0=w+13\quad 0=w−ix\) Since \(w\) is the width of the bedroom, it does not
make sense for it to be negative. We eliminate that value for \(due west\).\(\cancel{w=−xiii}\) \(\quad due west=9\) \(w=9\) The width is 9 feet. Discover the value of the length. \(w+4\)
\(nine+4\)
13 The length is 13 feet.Stride half-dozen. Check the answer.
Does the answer make sense?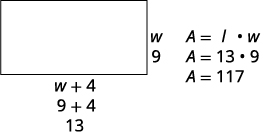
Yep, this makes sense.Step 7. Answer the question. The width of the chamber is nine feet and
the length is thirteen feet.
A rectangular sign has area xxx square feet. The length of the sign is i pes more than the width. Find the length and width of the sign.
- Answer
-
The width is 5 feet and length is 6 feet.
A rectangular patio has area 180 foursquare anxiety. The width of the patio is three feet less than the length. Find the length and width of the patio.
- Answer
In the adjacent example, we will apply the Pythagorean Theorem \((a^2+b^2=c^2)\). This formula gives the relation between the legs and the hypotenuse of a right triangle.
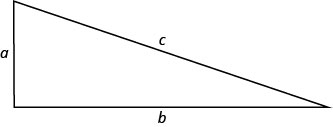
We will use this formula to in the next example.
A gunkhole's canvass is in the shape of a correct triangle as shown. The hypotenuse will be 17 anxiety long. The length of one side will exist 7 feet less than the length of the other side. Discover the lengths of the sides of the sail.
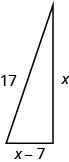
- Answer
-
Step 1. Read the problem Step 2. Identify what you are looking for. We are looking for the lengths of the
sides of the sheet.Step iii. Name what you are looking for.
One side is seven less than the other.Let \(x=\text{ length of a side of the sail}\).
\(ten−vii=\text{ length of other side}\)Step 4. Translate into an equation. Since this is a
correct triangle we can use the Pythagorean Theorem.\(a^2+b^2=c^2\) Substitute in the variables. \(ten^two+(x−seven)^2=17^2\) Step 5. Solve the equation
Simplify.\(x^2+x^2−14x+49=289\) \(2x^2−14x+49=289\) It is a quadratic equation, and so get zero on one side. \(2x^2−14x−240=0\) Factor the greatest common factor. \(two(x^2−7x−120)=0\) Factor the trinomial. \(2(x−15)(x+8)=0\) Use the Cipher Product Property. \(2\neq 0\quad x−15=0\quad x+8=0\) Solve. \(2\neq 0\quad ten=15\quad x=−eight\) Since \(x\) is a side of the triangle, \(ten=−eight\) does non
brand sense.\(2\neq 0\quad x=15\quad \abolish{ten=−eight}\) Detect the length of the other side. If the length of one side is
so the length of the other side is


8 is the length of the other side.Footstep 6. Check the answer in the problem
Exercise these numbers make sense?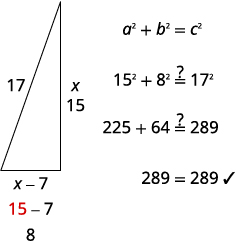
Step 7. Respond the question The sides of the canvass are eight, 15 and 17 anxiety.
Justine wants to put a deck in the corner of her backyard in the shape of a right triangle. The length of one side of the deck is 7 feet more than the other side. The hypotenuse is thirteen. Find the lengths of the two sides of the deck.
- Answer
-
v feet and 12 feet
A meditation garden is in the shape of a right triangle, with ane leg 7 anxiety. The length of the hypotenuse is one more than the length of the other leg. Find the lengths of the hypotenuse and the other leg.
- Answer
-
24 feet and 25 anxiety
The next example uses the office that gives the peak of an object as a role of time when it is thrown from fourscore feet higher up the ground.
Dennis is going to throw his condom band ball upward from the top of a campus building. When he throws the rubber band brawl from 80 feet higher up the ground, the function \(h(t)=−16t^2+64t+lxxx\) models the summit, \(h\), of the ball above the ground as a role of time, \(t\). Find:
ⓐ the zeros of this function which tell u.s. when the ball hits the ground
ⓑ when the ball volition be lxxx feet in a higher place the ground
ⓒ the tiptop of the ball at \(t=2\) seconds.
- Answer
-
ⓐ The zeros of this function are found past solving \(h(t)=0\). This will tell us when the ball will hit the footing.
\(\begin{array} {ll} &h(t)=0 \\ \text{Substitute in the polynomial for }h(t). &−16t^ii+64t+80=0 \\ \text{Factor the GCF, }−16. &−16(t^2−4t−5)=0 \\ \text{Factor the trinomial.} &−sixteen(t−5)(t+1)=0 \\ \begin{array} {l} \text{Use the Zero Product Holding.} \\ \text{Solve.} \terminate{array} &\begin{array} {ll} t−5=0 &t+1=0 \\ t=v &t=−1 \cease{array} \stop{array} \)The result \(t=v\) tells us the ball will hit the basis five seconds after it is thrown. Since time cannot be negative, the outcome \(t=−1\) is discarded.
ⓑ The ball will be eighty feet above the ground when \(h(t)=fourscore\).
\(\begin{array} {ll} &h(t)=80 \\ \text{Substitute in the polynomial for }h(t). &−16t^ii+64t+80=fourscore \\ \text{Subtract eighty from both sides.} &−16t^2+64t=0 \\ \text{Gene the GCF, }−16t. &−16t(t−4)=0 \\ \begin{assortment} {l} \text{Use the Zilch Production Holding.} \\ \text{Solve.}\end{array} &\begin{array} {ll} −16t=0 &t−iv=0 \\ t=0 &t=4 \terminate{array} \\ &\text{The ball will be at eighty anxiety the moment Dennis} \\ &\text{tosses the ball and then iv seconds later, when} \\ &\text{the brawl is falling.} \terminate{assortment} \)ⓒ To find the height ball at \(t=2\) seconds nosotros notice \(h(2)\).
\(\begin{array} {ll} &h(t)=−16t^2+64t+80 \\ \text{To find }h(2)\text{ substitute }2\text{ for }t. &h(two)=−xvi(ii)^ii+64·2+80 \\ \text{Simplify.} &h(2)=144 \\ &\text{Afterwards 2 seconds, the ball will be at 144 feet.} \cease{assortment}\)
Genevieve is going to throw a rock from the top a trail overlooking the ocean. When she throws the rock upward from 160 anxiety above the sea, the function \(h(t)=−16t^ii+48t+160\) models the height, \(h\), of the stone higher up the ocean as a role of time, \(t\). Find:
ⓐ the zeros of this function which tell us when the rock will striking the body of water
ⓑ when the rock will be 160 feet in a higher place the sea.
ⓒ the height of the rock at \(t=ane.five\) seconds.
- Answer
-
ⓐ five ⓑ 0;three ⓒ 196
Calib is going to throw his lucky penny from his balcony on a cruise ship. When he throws the penny upward from 128 feet above the ground, the function \(h(t)=−16t^2+32t+128\) models the superlative, \(h\), of the penny above the bounding main as a function of time, \(t\). Find:
ⓐ the zeros of this role which is when the penny will hit the ocean
ⓑ when the penny will be 128 feet above the ocean.
ⓒ the height the penny will exist at \(t=ane\) seconds which is when the penny volition exist at its highest indicate.
- Respond
-
ⓐ 4 ⓑ 0;2 ⓒ 144
Access this online resource for additional didactics and practise with quadratic equations.
- Beginning Algebra & Solving Quadratics with the Cipher Property
Central Concepts
- Polynomial Equation: A polynomial equation is an equation that contains a polynomial expression. The degree of the polynomial equation is the degree of the polynomial.
- Quadratic Equation: An equation of the form \(ax^2+bx+c=0\) is called a quadratic equation.
\[a,b,c\text{ are real numbers and } a\neq 0\nonumber\]
- Cypher Product Property: If \(a·b=0\), and then either \(a=0\) or \(b=0\) or both.
- How to use the Zero Product Property
- Set each gene equal to null.
- Solve the linear equations.
- Check.
- How to solve a quadratic equation by factoring.
- Write the quadratic equation in standard form, \(ax^2+bx+c=0\).
- Cistron the quadratic expression.
- Use the Zero Product Property.
- Solve the linear equations.
- Cheque. Substitute each solution separately into the original equation.
- Nothing of a Function: For any office \(f\), if \(f(x)=0\), then \(x\) is a null of the role.
- How to use a problem solving strategy to solve give-and-take problems.
- Read the problem. Make sure all the words and ideas are understood.
- Place what we are looking for.
- Name what nosotros are looking for. Choose a variable to correspond that quantity.
- Translate into an equation. It may exist helpful to restate the problem in one sentence with all the important data. And then, interpret the English sentence into an algebraic equation.
- Solve the equation using appropriate algebra techniques.
- Check the answer in the problem and make sure it makes sense.
- Answer the question with a complete judgement.
Source: https://math.libretexts.org/Courses/Las_Positas_College/Foundational_Mathematics/14%3A_Factoring/14.06%3A_Polynomial_Equations
0 Response to "Quadratics and Polynomials Word Problems Review Question 6 Answers"
Post a Comment